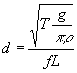

Put a bending fixture on an outdoor camping stove, then one end of the
bentside board is clamped on the fixture and the other end is pulled down
to give a proper curvature. To bend the board for a large area, the clamping
position can be repeatedly moved as needed. Bending compliance of the board
cannot be homogeneous and the board can be slightly twisted after removing
the force and the temperature. If it happened, the pulling force at the
both sides of the board can be independently adjusted. Using an industrial
heat-gun, locally applying additional heat is sometimes effective for correcting
the twist. Careful management of temperature is necessary not to burn the
board.
The photo on the left is showing the bending process for a double bentside
of a Germann harpsichord.






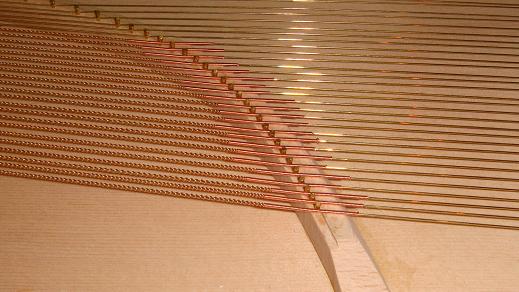
Over-spun wire
When a higher wire tension is required, either longer speaking length,
thicker wire or heavier materials can be employed. Brass strings, having
heavier specific gravity than iron, need to be stretched with higher tension
to achieve the same pitch. If materials heavier than brass is used, instruments
can have strings with even higher tension. However, from the practical
point of view , no materials other than brass or other copper alloy can
be found. It is difficult to have harmonics rich sound with too thick strings.
Then, an over-spun wire, is used. It provides heavier effective density
by winding a soft and heavy wire over the core string.
String tension can be calculated with pitch(frequency), speaking length
and weight per unit length of the wire. The weight per unit length is calculated
with diameter and bulk density of the wire. It is convenient to use an
effective weight per unit length calculated with an apparent bulk density
of the over-spun wire. The formula to give the apparent density can be
found here.

A hand drill is used as a power source. The lever in the front is a power
switch. Two individual gear boxes, located at right and left ends, are
connected with a hexagonal bar.

The left gear box, connected with a hexagonal bar, is fixed on a workbench
with a clamp. The left gear box can be clamped at any position according
to the wire length. The clamp is also convenient to apply tension to the
core wire.

Where the tangent hit the overspun wire, the cover wire is tightly winded
and the tip of the tangent is bent to prevent any damages on the cover
wire.
Where the overspun wire touches with the bridge pin, the cover wire is also tightly winded. This is to prevent any damage of cover wire when the bridge pin rubs the cover wire while tuning or putting new strings.






A magnetic device to measure sound board thickness
Assuming a detailed explanations of how the device works is not widely
available, though sometimes it is referred as "magnetic method",
a schematic drawing, showing the principle of the measurement, is provided
below. Measurement accuracy is not as good as those with dial gauges.
However, the accuracy can be statistically improved by measuring several
times and average them.
The schematic drawing shows only the principle. The blue arm in the
left is slowly pulled-up. As soon as the magnet, attached to he coil spring,
separates from the board, read the thickness of the board, indicated
on the scale (red colored). Another magnet below the board still stays
there. The blue arm needs to be pulled more, if the board is
thinner.

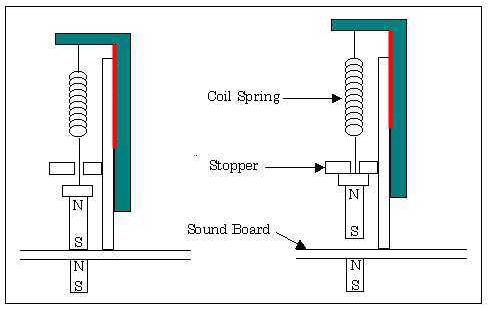
When the outer case, which has a scale, is pulled up, the coil spring is
streched and upword force is applied to the magnet, above the soundboard
material. When the upword force becomes greater than the magnetic attraction
force with another magnet under the board, the upper magnet comes apart
from the board. At this moment, the scale indicate the thickness of the
board.






Wire Tension
Wire tension can be calculated with following formulas.
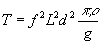 ,
, 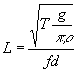 ,
, 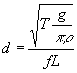
where:
T: Tension
L: Speaking length
d: Diameter of the wire
f: Resonance frequency
r: Bulk density of the wire material
Apparent density of wound wire can be calculated, using below formula:
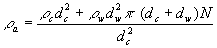
where:
ra: apparent bulk density for tensile load
rc: bulk density of the core wire material
rw: bulk density of the cover wire material
dc: diameter of the core wire
dw: diameter of the cover wire
N: number of wind per unit length
By pressing below buttons, calculated results of string tension for each
of the instruments shown in this web site are available.
German Harpsichord 8feet German
Harpsichord 4 feet
Italian Harpsichord
Italian Virginal
French Harpsichord 8 feet
Flemish Harpsichord 8feet Flemish Harpsichord 4
feet
Flemish
Virginal
Square
Piano
Fretted Clavichord Unfretted Clavichord
Geigenwerk Geigenwerk2 Streichklavier






Key touch:
To play a clavichord, a player is required to hold the strings after the
tangent hits the strings. The elastic key touch to further hold the strings,
after the sound released, is the source of musical expressions specific
to clavichords in addition to controlling the speed of tangents to hit
the strings.
In the sharp keys, due to short distance between the balance pin and the
key front, the key lever acts as a hard lever. For example, with the same
string tension and the same striking point, a player needs stronger finger
pressure to hold the strings for sharp keys than those for natural keys.
In a fretted clavichord, for example, c and c# share the same pare of strings.
The c# striking point is closer to the center of the string and the force
necessary to hold the string at the tangent is smaller than that for c.
In a fortunate case, this soft force at the tangent is combined with the
hard lever of c#, the finger pressure necessary to hold the c# string can
be well balanced with the force for c. However, in the case of b♭(e♭)
and b (e), the unbalance is magnified.
Individually balancing the key touch can be assumed to be one of the advantages
of unfretted clavichords. The key touch is determined with following three
parameters namely, string tension, striking point and key lever. Respecting
the available data of original design for striking point and key lay out,
adjustment can be made with selection the proper string gauges in order
to realize smooth changes in the key hardness.
In the beginning, the string gauges were selected to provide smooth changes
in the string tension over the whole range of the compass. However, a significant
difference in key hardness between low-mid range and high range was found.
Then, string gauges were reselected to provide smooth change in the key
hardness.
For each note, string thickness is selected in order to fit with predetermined
string tension. Then, after a tangent touches a pair of strings, force
necessary at the key front to further push up the tangent for 2mm is calculated,
using data of striking point and length between balance-pin/tangent and
key-front/balance-pin. The first two figures represent key touch weight
of fretted clavichords of Donat and Hubert models and the later two figures
are for unfretted clavichords of Hoffmann and Silbermann models.
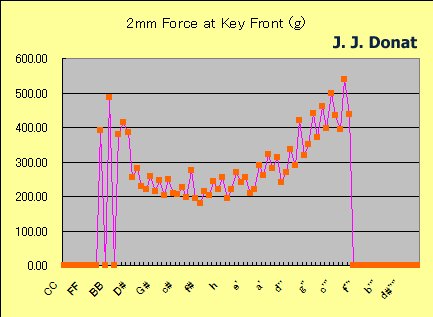
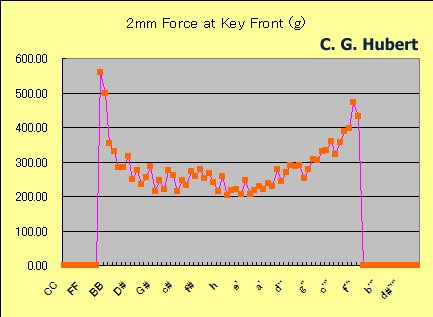
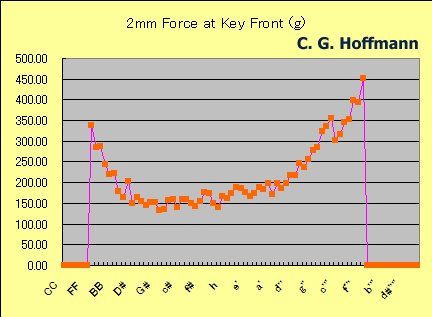
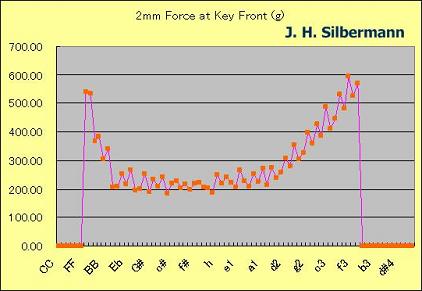





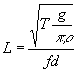 ,
,